In quantum mechanics, a two-state system (also known as a two-level system) is a quantum system that can exist in any quantum superposition of two independent (physically distinguishable) quantum states. The Hilbert space describing such a system is two-dimensional. Therefore, a complete basis spanning the space will consist of two independent states. Any two-state system can also be seen as a qubit.
Two-state systems are the simplest quantum systems that are of interest, since the dynamics of a one-state system is trivial (as there are no other states the system can exist in). The mathematical framework required for the analysis of two-state systems is that of linear differential equations and linear algebra of two-dimensional spaces. As a result, the dynamics of a two-state system can be solved analytically without any approximation. The generic behavior of the system is that the wavefunction's amplitude oscillates between the two states.
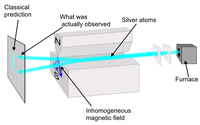
A very well known example of a two-state system is the spin of a spin-1/2 particle such as an electron, whose spin can have values +ħ/2 or −ħ/2, where ħ is the reduced Planck constant.
The two-state system cannot be used as a description of absorption or decay, because such processes require coupling to a continuum. Such processes would involve exponential decay of the amplitudes, but the solutions of the two-state system are oscillatory.
YouTube Encyclopedic
-
1/5Views:19 24916 3732 5297 6248 704
-
16. Quantum Dynamics (continued) and Two State Systems
-
17. Two State Systems (continued)
-
Pure VS Mixed States | Quantum Information
-
36. Time Dependence of Two-Level Systems: Density Matrix, Rotating Wave Approximation
-
L24.2 The simplest quantum system.
Transcription
The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation, or to view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu. PROFESSOR: OK, today's lecture will begin with photon states, which is a very interesting application of what we've learned about coherent states. And in a sense, it's an advanced topic. Photon states are states of the electromagnetic field. They are quantum states of the electromagnetic field. You A photon, this particle, is a quantum of the electromagnetic field. There's a discrete piece of energy and momentum carried by this particle. So when we talk about photon states, we're really doing quantum field theory. So in some sense, this lecture, you will see how quantum field theory works. A first introduction to quantum field theory. And it's interesting that the harmonic oscillator plays such an important role in that. So a key identity that we are going to use, of course, is this coherent states that were defined as displacements of the vacuum. For D, if I remember right, was e to the alpha a dagger minus alpha star a. And it had the property that a acting on alpha was equal to alpha-- alpha, the operator a. So these were the coherent states we've been talking about. And today we're going to talk about photon states. So that will be probably about half of the lecture. And in the second half of the lecture, we will begin a more systematic study of two-state systems. Two-state systems, of course, are our spin states, are the classical two-state system. And we're going to sort of put it all together. We'll understand the general dynamics of a two-spin system, what is the most general Hamiltonian you can have, and therefore the most General Dynamics. And then we'll solve that. And we'll have two physical examples, one having to deal with the ammonia molecule. And another having to do with nuclear magnetic resonance. Both are applications of two-state systems. So till it's the end of the lecture, we'll be doing that. So about photon states. Well, photon states have to do with electromagnetic fields. That's electric and magnetic fields. And one important quantity that you know about the electromagnetic fields is the energy. If you have an electromagnetic field, you have an energy. And remember, energies have to do with Hamiltonians. So we're going to try to do a quantum description of the electromagnetic field. Therefore, knowing the energy would be a good place to start. So the energy, as you know, in an electromagnetic field goes like e squared times some epsilon and b squared. And you add the two of them. So here we go. Let me write a precise formula. The energy is equal to 1/2 the integral over volume, epsilon 0, the electric field squared, plus c squared times the magnetic field squared. So this is our energy. And we're going to try to describe a configuration of electromagnetic fields. We will to focus on one mode of the electromagnetic field. So I will imagine I have some sort of cavity, finite volume. And in there I have one electromagnetic field, what you usually called in 802 or in 8022 or 807 a wave. A single wave with some wavelength, some frequency, and that's all we have. So we're going to simplify to the case where we have a single one consistent with Maxwell's equations and some boundary conditions that we need not worry about. And I will normalize them as follows with a V in here. That this is the volume of the system. So, volume. And that could be the volume of the cavity that has this electromagnetic field. Or some large box. Or you can let it almost be infinite and work with that as well. So we'll have a wave. Omega would be the frequency. K is omega over c for a electromagnetic wave. So we'll have this times omega sine of kz, a spatial distribution. And there will be a function of time, as you know. But this function of time, I want to leave it a little ambiguous at this moment-- or, general. Not ambiguous, general. So I'll call it q of t, some function of time to be determined. There's going to be an electromagnetic and magnetic component to this field, By. c times By will also depend on z and t and will have the same pre-factor. I put the c here. So your c squared b squared also works well. Epsilon 0 v. This time I'll put another function, p of t cosine of kz. It's another function of time and I just call them that way. There is a question there. STUDENT: Why is your frequency outside your function of time? PROFESSOR: It's just another constant here. STUDENT: What would that mean then? PROFESSOR: No particular meaning to it. At this moment, whatever this constant is you would say probably it's useful because you somehow wanted the q here. That has some meaning. So you probably would put the same constants here in first trial. You wouldn't have this omega here. But if you put it, this is just another way of changing their own manifestation of q. So it doesn't have a profound meaning so far. Any other questions about this? This is and electromagnetic field configuration. And this q of t and p of t are functions of time. You know your Maxwell's equations. And you will check things related to Maxwell's equations for this configuration in the homework. But at this moment, it's not too crucial. The thing that this important is that we can try to calculate the energy now. And if you do it, well, the squares, the epsilon 0's are going to disappear. And you're going to have to integrate over the box, this integral of sine squared of kz or cosine squared of kz. The functions of time don't matter-- this energy could depend on time. And the way we've prepared is when you integrate over sine squared of kz, if the box is big, it's a good situation where you can replace that for 1/2, which is the average, and 1/2 for the average of this. Or you could define where the box extends, from what values of z to what other values of z's. And so the integral, in fact, is not any complicated integral. And we have immediately the answer that energy is 1/2 p squared of t plus omega squared q squared of t. And that was why this omega was here. There's not really much to this. Except that when you square it and you take the integral over the volume, you replace the sine squared by 1/2 and the cosine squared by 1/2. And that's it. So actually, the labels that we've chosen here are pretty good. This starts to look like a harmonic oscillator. Except that the mass is gone. 1 over 2m p squared should be plus 1/2 m omega squared q squared. So the units are wrong here. p squared over 2m has units of energy. But p squared doesn't have units of energy. And 1/2 m omega squared q squared has units of energy but this one doesn't. So the units are a little off for a harmonic oscillator. So it's interesting to notice now. But you couldn't have done better. Because photons have no mass. And we're trying to describe the electromagnetic field. It has photons. So there's no way this could have showed up a mass there. There's no such thing. And that's why it doesn't show up. On the other hand, you can say, well, OK, let's see if this makes a minimum of sense. How do we deal with this unit? So p has units of square root of energy. And q has units of time times square root of energy. Why is that? Because omega has units of 1 over time. So q over time squared is energy. So q is t times square root of energy. And therefore p doesn't have the right units to deserve the name p. And q doesn't have the right units to deserve the name q. But p times q has the units of time times energy, which are the units of h bar. So that's good. This p and q have the right units in some sense. So this thing could be viewed as an inspiration for you. And you say at this moment, well, I don't know what is a quantum of an electromagnetic field. But here I have a natural correspondence between one mode of vibration, classical, of the electromagnetic field, and an energy functional that looks exactly like a harmonic oscillator. So I will declare these things to be a Hamiltonian and this p of t and q of t to be the Heisenberg operators of the electromagnetic field. So what we're saying now is that I'm going to just call the Hamiltonian 1/2 p hat squared plus omega squared q hat squared. This is a time independent Hamiltonian. If you're doing Heisenberg, it's the same thing as the Hamiltonian that would have p hat square of t plus omega q hat squared of t. Now, at this moment, this might sound to you just too speculative. But you can do a couple of checks that this is reasonable. So one check, remember that the Hamiltonian-- quantum equations of motion, of Heisenberg operators should look like classical equations of motion. So I can now compute what are the Heisenberg equation of motions for the operators. Remember something like v dt of p Heisenberg of t dt is related to h with p Heisenberg. And you can calculate the Heisenberg equations of motion. I may have signs wrong here. Nevertheless, you know those for the harmonic oscillator and you can write them. But you also know Maxwell's equations. And you can plug into Maxwell's equations. And that's one check you will do in homework, in which you will take Maxwell's equations and see what equations you have for q of t p of t. And then they will be exactly the same as the Heisenberg equations of motion of this Hamiltonian, giving you evidence that this is a reasonable thing to do. That we can think of this dynamical system with q and p being quantum operators. So let's accept that this is a Hamiltonian for this quantum system that we want to work with. And therefore, write the operators that we have. And what are they? Well, we had formulas with masses. But now mass goes to 1. So know the units. You cannot let in general in a formula mass going to 1 unless you're going to do something with the units. But we agreed already that these p's and q's have funny units. So those units are in fact consistent with a mass that has no units. And you can set it equal to 1. So I claim that you can take all the formulas we had with m and just put m equals to 1 and nothing would go wrong. Nothing goes funny. So in particular, you had an expression for x that now is called q terms of creation and annihilation operators and now that reads-- And you have an expression for p. And that one reads now a minus a dagger. These formulas used to have m's in there. And I've just set m equals to 1. And that should be the right thing. Unit-wise, indeed h bar omega has units of energy. And we claim that p has units of energy, square root of energy. So this is fine. So what else do we get from this Hamiltonian? Well, we can write it in terms of the number operators. So this Hamiltonian now, it's equal to h bar omega a dagger a plus 1/2. And this is just because this p and q written in this way corresponds to m equals to 1. And m doesn't show up anyway in this formula. So no reason to be worried that anything has gone wrong. And this is H equals to h bar omega, N hat plus 1/2. And this is a number operator. And then you get the interpretation, the physical interpretation that if you have states with some number operator, the energy is the number times h omega, which is exactly what we think about photons. If you have N photons in a given state, you would have an energy N times h bar omega. So it may look a little innocent what we've done here. But this is a dramatic assumption. You've really done something that took physicists 30 years to figure out, how to do quantum field theory. And of course, this is just the very beginning. And there's lots of things to learn about it. But the first thing that is happening is that somehow-- look what's happening. In normal quantum mechanics, x and p became quantum operators. In a sense here, this q and p are like x and p. But they have nothing to do with usual position and momentum. Nothing absolutely. q is like E really. And p is like B. So who has become a quantum operator? Not x and p, in a sense. E and B have become quantum operators. Quantum field theory is the idea that the fields become operators. That's what's really happening. And it seems to be right in the sense that our intuition that the state with N photos would be viewed as a state of a harmonic oscillator, an usual one with mass equals 1. So that this really is not a momentum and this is not a position. But they behave as that. So we can turn now this formula to its Heisenberg form so that q of t is square root of h bar over 2 omega. Remember a as a function of time becomes e to the minus i omega t a hat-- that's the Heisenberg version of a-- plus e to the plus i omega t a hat dagger. So given that, we can substitute back to our electric field that has this omega here, that has this factor in there. So I will write it all together here. Therefore, Ex of z t-- and now I've put a hat here. And z t, the t is the t of a Heisenberg operator now. Is equal to E naught e to the minus i omega t a plus e to the i omega t a hat dagger sine of kz, where this constant E zero is h bar omega over epsilon 0 V. It's just a mnemonic for some constant at this moment. So we plugged-in here, there's all these factors. There's the omega and there's the q. So all these factors together give you this. The factor and sine of kz. And this is the electromagnetic field operator. The electric field is not anymore an electric field. It's an operator. So if we want to get an intuition about this electric field operator, let's try to find its expectation value. It's an operator. The closest thing we can have an intuition about an operator is its expectation value. So very good. Let's take a photon state and energy eigenstate of the harmonic oscillator of occupation number n. And we have a state now of n photons, an energy eigenstate. In fact, with energy n h omega plus this 1/2 h bar omega. And let's figure out what is the expectation value of Ex in that state n. So we go to this formula. And we say, OK, it's E naught e to the minus i omega t n-- and we're all very curious. We want to see how the electromagnetic field of the n-th state of the harmonic oscillator, n photons in an energy eigenstate, how does that wave look? Let's see. n a hat n plus e to the i omega t n a dagger n sine kz. So this is a field operator. So we put it in a state and we want to know how does the field look in that state. And how much do we get? STUDENT: [INAUDIBLE]. PROFESSOR: 0. OK, that seems a little strange. Because indeed, the matrix element of a in an energy eigenstate is 0. This reduces, makes n minus 1 [INAUDIBLE] to this. So this is 0. And this is n plus 1 n. This is 0. So actually no great illumination nation has happened. We got 0. So actually this is not too strange. Energy eigenstates are very unintuitive. The energy eigenstate of a harmonic oscillator, the n-th state is some sort of wave that is like that. Nothing changes in time in that wave. Nothing all that interesting happens. So the fact that this electromagnetic field operator has zero expectation value on this n photon state is maybe not too surprising. So let's take a more thoughtful state. We've said so many times that coherent states act like classical states. So let's put a coherent state of photons into this state. So let's see. Now the state will be an alpha state, which is a coherent state. And therefore, the expectation value of Ex on the alpha state will be equal to E not e to the minus i omega t alpha a alpha plus e to the plus i omega t alpha a hat dagger alpha sine of kz. Well, we're in better shape now. a on alpha is the number alpha, as we reviewed at the beginning. And then alpha with alpha is 1. Remember, it's a unitary transform of the vacuum. Therefore, this whole thing is alpha. So this is E not alpha being a number e to the minus i omega t plus here is alpha star e to the i omega t sine of kz. And now we're very happy. The coherent state is the state for which the expectation value of the electromagnetic field is precisely the kind of waves you've seen all your life. This wave, travelling waves, stationary waves. All those only appeared because only on coherent states a and a dagger have expectation values. So what we really call a classical wave resonating in a cavity is a coherent state of the electromagnetic field in this sense. The state of photons form a coherent state. They're not an energy ion state. They're not positioned for anything. They're not the number eigen states either, because they're not energy eigen states. They have uncertainties. But they have a nice, classical picture. The expectation value of the operator is a real wave. So any time in 802 or in 8022, you have a classical wave to analyze, the quantum description of that wave is a coherent state of the electromagnetic field. Lasers are coherent states of the electromagnetic field. They have these uncertainties that we discussed last time with number and phase that are very strong. If the number goes large, then certainty on the face is extremely small. So there we go. This is a coherent state. We can do a little more on that, write it more explicitly. This is epsilon 2 E not, the real part of alpha e to the minus i omega t sine of kz. And if we write, for example, alpha to be length of alpha e to the i theta, then this would be 2 E not. Length of alpha would go out, and the i theta to minus i omega t would give you cosine of omega t minus theta sine of kz. And this is something like a standing wave. It just changes in time and with a fixed spatial distribution. So it's a classical wave, and nevertheless, it has a good description classically, a good description quantum mechanically. It's a coherent state. And its energy is the expectation value of the Hamiltonian. The expectation value of the energy-- let me write this expectation value-- of H is H omega. Expectation value of N plus 1/2. And in a coherent state, the expectation value of N is alpha squared. So you have this of the coherent state alpha has alpha squared photons. And that's because it's the number operator, and that's pretty much the end of our story for photon states. There's more that one could do. One could do basically all kinds of things put together different modes. We considered here one mode. You could consider electric fields have super procession of modes and discuss commutation relations for the field operators, and all kinds of things. But that's really a quantum field theory course. At this moment, the main story I wanted to get across is that naturally, the harmonic oscillator has entered here, but in a very funny way. q and p were not positioned in momentum, were basically electric field and magnetic field. And there's an uncertainty between electric and magnetic fields. And the result of all this is that at the end of the day, you have a description by a harmonic oscillator and with energy levels that correspond to different amount of photons in the field. Finally, the classical things, if you want to recover classical waves, you must consider coherence states. These are the states that were classical. When you looked at the harmonic oscillator doing motion and for electromagnetic field, they give you the classical wave picture of an electric and magnetic field oscillating in position and time. So are there any questions? Yes. AUDIENCE: If we're associating h bar with [INAUDIBLE], what object would you associate the zero point energy with? PROFESSOR: Well, it's a zero point energy of this quantum of vibration. So just like an electromagnetic field, basically, if this is like q and p, there's a minimum energy state in which you're in the ground state of the harmonic oscillator. But E and B cannot be zero, like delta x and delta p cannot be zero. So every mode of the electromagnetic field has a zero point energy. You cannot reduce it. So the vacuum of the electromagnetic field has a lot of zero point energies, one for every mode of radiation. Now, that zero point energies don't get you in trouble unless you're trying to do gravity. Gravity's the universal force and universal interaction that notes every bit of energy. So your zero point energies are quite important if you consider gravity. And you would have encountered here the first complication associated with quantum field theory. Every mode of the electromagnetic field-- a frequency one, a frequency 1.1, a frequency 1.2-- every one of them has a ground state energy of 1/2H bar omega. If you add them all up, you get infinity. So you get an infinity of ground state energies. And people have learned how to work with this infinities. That infinity is not physical. But, if you suitably treat it, you can figure out all kinds of things. And there's several people, I think even some undergraduates, working on this with Professor Kardar and Professor Jaffe, called Casimir energies, in which the zero point energies of the electromagnetic field are treated in a more careful way, and the infinities are seen to be irrelevant, but there are some physical dependence on the parameters that keeps there. So you see the origin of this is because every mode of the electromagnetic field has a zero point energy, just like any quantum oscillator. Yes. AUDIENCE: [INAUDIBLE]. PROFESSOR: Absolutely. AUDIENCE: [INAUDIBLE]. PROFESSOR: Well, uncountable things, we already have seen some. Maybe they didn't look that sophisticated, but we had position states that were uncountable. So the electromagnetic field, yes, it has uncountable things. And there's nothing wrong about it. You just have to work with integrals. AUDIENCE: [INAUDIBLE]. PROFESSOR: Well, no, no. They're not really normalized because just like these states, the position states are not normalized, they're delta function normalized and things like that. So look, if you want to avoid conceptual troubles with that, people and many physicists and textbooks on quantum field theory begin with space, a big, big, box. And then you see that it works for any size box, and then you say, well, it will work if the box is infinite. And we just proceed. All right. So I'll move on now to the second part of the lecture that deals with two-state systems and spin states and goes back and puts together a few of the things we've been doing. AUDIENCE: Professor? PROFESSOR: Yes. AUDIENCE: Could you close the sun shade? I can't really see the board. PROFESSOR: OK, sure. Board. I think maybe we need all the way? No, that won't make a difference. It's the other shades, I think. I'll leave it like that. Maybe I should use another board for the people that watch these movies. That may be better. So let's do this board. OK, so here's what we want to understand. Two-state systems. It's probably going to be about this, and two more lectures on that. And what we want to understand first is spin procession. You say, well, spin procession looks like a very particular kind of problem. When you have spins, you have magnetic fields. But at the end of the day, what we will see is that spin process-- you can view any two-state system as a system in which you've put a spin in a magnetic field. Even though you may be talking about electrons shared between two atoms, or ammonia molecule, or anything like that. Mathematically, you go back always to spins. Because spins are things we have become familiar already. So we exploit that to the maximum. So we do the one thing we haven't done rigorously so far, and then we'll explore this analogy to some point. So what was our discussion of spin? So two-state systems, and we'll begin with spin procession. So the idea of spin procession all arises, as you remember, because if you have a charged particle that has some spin, there's a relation between the particle's magnetic moment and the spin, or the angular momentum, of that particle, of that little ball of material. And we made an argument that this was just q over 2m times the angular momentum. And this will be angular momentum. This was classical. Nevertheless, the fact that we claim is true quantum mechanically is that in fact this idea is roughly right, except that there's two modifications. The true magnetic moment that enters into the Hamiltonian under the particle has is not quite the same as suggested by the classical argument, but it's modified by a g factor. And that modification is important. And this S is not just a plain classical angular momentum of a rotated ball with some mass and some radius, but it's a spin angular momentum and intrinsic angular momentum. A rather abstract thing that in fact should be best viewed as an operator, and that's the way we've thought about it. The magnetic [INAUDIBLE] now becomes an operator, because it's proportional to the spin operator. So it's an operator. And different values of g apply for different particles. And we saw that g equals 2 applies for the electron. That's a famous value, in fact predicted by Dirac's equation, relativistic equation, for the electron, and observed to great accuracy of course as well. And for other particles, like the proton or the neutron, the quantity g has different values. You might be surprised that the neutron has a dipole moment. Because you would say a neutron is an uncharged particle, so a charge rotating doesn't do anything. Nevertheless, a neutron is uncharged, but it has three quarks, two with some charge, one with an opposite charge to the other two. And if they distribute cleverly, say the negative ones are farther away from the center, and in the center is the positive one, this could have angular magnetic moment. And in fact, it does have magnetic moment. The neutron has a significant magnetic moment. So at the end of the day, we're going to write this as mu equal gamma S. And this constant gamma is going to summarize everything, g, q, m, all these things. And this will be a good notation. Gamma S is brief and simple. And this constant, we're going to use it. So the Hamiltonian minus mu dot B is a quantum Hamiltonian because mu is an operator. B, at this moment, even though we were just talking about photon states, this will be a static magnetic field typically. Can be a to time dependent, but it will not be sufficiently important if it has time dependence, and we have to quantize it and to think of it as a quantum field. But in some problems of radiation of electromagnetic fields by the motion of spins, you would have to quantize the electromagnetic field. But this is not the case now. So this is minus gamma S dot B. And we typically like to write it as minus gamma B dot S. And that means very explicitly minus gamma BxSx operator plus BySy operator plus BzSz operator. So let me remind you of a simple situation when you had a magnetic field in the z direction. B along z if B is B times z hat. Then H is minus gamma. BSz. And the unitary operator that generates time evolution of states, the unitary operator u of t0 is exponential minus i. I'll call it H sub s for spin. H sub s t over H bar. And I'll put it like this exponential of minus i minus gamma B t Sz over H bar. So I substituted what Hs is, moved the t sort of inside the parentheses minus gamma B Sz. I put the z out and put this here. So far so good? This is our time development operator. Now, I want you to recall one property that you only justified by checking it in the homework. But in the next few lectures, we will just make sure you understand this why it's true in general. But we talked in the homework about an operator Rn sub alpha, which was exponential of minus i alpha Sn over H bar. Where n was a unit vector, and Sn is defined as n dot S. So nxSx, nySy, nzSz. So this operator that you considered was called the rotation operator, and it did perform rotation of spin states. In fact, what it did was rotate any spin state by an angle, alpha, around the nth direction. So if you had the n direction here, and you had any spin state in some arbitrary direction, it would rotate it by an angle alpha around this. So you have this, it would rotate it to another point over here with an angle alpha in between. So in words, it rotates by an angle alpha, rotates spin states. And when you think of a spin state, you must think of some n vector, n prime vector. So maybe n prime here would be a good notation. So you have a spin state in the n prime direction. Remember your spin states were of the form n plus minus. Well, the state that points in the direction n is n plus, so some n prime direction. This operator rotates those states by an angle alpha. Now, it probably is a little vague in your mind, that idea, because you checked it several weeks ago. And you only checked it by taking some particular states and rotating them. So we will have to elaborate on this, and we will. So this will become clear that this rotates any spin state by an angle alpha and rotates spin states using an axis, with respect to the axis defined by n over here. So that's interpretation of this state, of this operator. That's what it does. And now I want you to look at this operator. Well, it's similar. In fact, this plays the role of alpha, and this plays the role of Sn. So this is the spin in the z direction, and this operator must rotate states by this angle alpha, which is gamma Bt. If what we said is right, that's what this operator must do. Even though I think you've done this calculation as part of tests, problems, or other problems, practice problems, not quite homework., I want to do this calculation again. So let's take an arbitrary spin state, xyz. Now, don't confuse the arbitrary spin states with the n here. The n is here the axis around which this Hamiltonian rotates states. But there's no states here. This is a rotation operator. I'm sorry, I called it a Hamiltonian. It's not precise. This is a unitary operator. It rotates states. And this is the direction, the axis, of rotation. Your spin state is another object. It's a spin that lives in some direction. So here, we're having the magnetic field in the z direction. So the magnetic field is here. And we'll put a spin state over here, an n, a spin state that has some value of phi and some value of theta. And that's the spin state at time equals zero. So psi 0 is the spin state this that with your formula sheet, this cosine theta over 2 plus plus sine theta over 2 e to the i phi, I think with a plus, yes. I'll call it phi not, and maybe theta 0, y 0, and minus. So this is a state, a spin state pointing in this direction, the direction n. That was the general formula for a spin state. Now we are going to apply the operator, the time evolution operator. But let's do a preliminary calculation. HS on plus is minus gamma B Sz on plus minus gamma B H bar over 2 plus, and Hs minus is equal to minus gamma BSz on minus equal plus gamma B H bar over 2 minus. So we want to add with this operator on this state. So here we have it, the state that any time is going to be E to the minus iHst over H bar times this state over here acting on psi is 0. So let's do it. Well, on the first term is cosine theta 0 over 2. And you have this exponent acting on plus. But the exponent has Hs that's acting on plus is this. So you can just put that thing on the exponent. So you put e to the minus i, and Hs on plus is this, minus gamma B H bar over 2. Then you have the p and the H bar and the plus. And continue here. So we just need to do the second term, plus sine theta over 2, e to the minus i. And now the same thing, but with a plus sign. Plus gamma B H bar over 2, t over H bar on the minus state. So just in case I got you confused and the small type is a problem here, this operator active on initial state just acts on plus, then acts on minus. On plus, the operator is an eigen state. So you can just put the number in the exponential. So you put the plus eigen value, the minus eigen value. So what do we get? Psi t is equal, cosine theta not over 2, e to the i gamma B t over 2 plus sine theta not over 2, e to the minus i gamma B t over 2 minus. Now, this state this is not quite-- I hope I got my signs right. Yes. This state is not quite in readable form. To compare it with a general end state, you need null phase here. So we must factor this phase out. e to the i gamma B t over 2. And it's an irrelevant phase. So then you have cosine theta not over 2 plus sine theta not over 2. I'm sorry, I forgot to have the e to the i phi not here. I didn't copy it. So here, what do we have? e to the i phi not minus gamma B t minus. Look, when you factor this one out, you get minus the same thing here. So this becomes a minus 1. And then you put the two faces together, and you got that. So now you look at this state, and you say, oh, I know what this is. This is a spin state that has theta as a function of time, just theta not. But the angle, phi, as a function of time is phi not minus gamma B t. So this spin will precess and will go like this. Phi not minus gamma B t is the phi as a function of time. So have the magnetic field. You have a procession of the spin over here. So this is spin procession. And indeed, this is exactly what we're claiming here. If this rotates states by an angle alpha, this operator, this Hamiltonian that we've discussed here, must rotate states by this angle alpha, which is minus gamma Bt, along the z-axis. So you have the z-axis, and you rotate by minus gamma Bt. The sine is the reason the phi decreases in time and goes in this direction, as opposed to going in the other direction. So this is a basic confirmation of what the spin is doing. And I want to give you the general result so that you can really use it more clearly. So I think the lights are gone, so we can go to this blackboard. First of all, classical picture. What is it about spin procession? Is it a quantum phenomenon or a classical phenomenon, or both? Well, it's really both. And this idea of procession, you can get it from the classical picture as well. So what do you have? If you have a mu in a B field, you get a torque. And that you can easily convince yourself. I'm sure you've done the computation in 802. You have a little square wire not aligned with the magnetic field. You calculate the force on one side, the force on the other. You see that there is a torque. And the torque is given by mu cross B. That's enm. On the other hand, the rate of change of angular momentum is the torque. So this is mu cross B. But mu is gamma S, so this is gamma S cross B. And this is minus gamma B cross S. OK. This equation, which I rewrite it here, ds/dt equals minus gamma B cross S, is a particular case of a very famous equation in classical mechanics, and this equation for a rotating vector. If you have a vector, dx/dt is omega cross x. This is the equation satisfied by a vector x that is rotating with angular frequency omega around the axis defined by the vector omega. A famous equation. OK, so you have here omega vector is omega n. So here is the direction of n, the unit vector. Here's omega. And you have a vector x over here. Then this vector, the solution of this equation, is a vector that is rotating around omega with the angular velocity magnitude of omega. In the notes, I just give a little hint of how you derive that. But truly speaking, you guys should be able to just scribble a few notes if you don't know the situation by heart, and convince yourself this is true. So this equation is of that form in which the omega x is played by S. Omega is minus gamma B. So this defines what is called the Larmor frequency, which is minus gamma B, is the Larmor frequency. Now, this Larmor frequency is precisely that one because was minus gamma B. And here you have minus gamma B times t. Omega times t is the angle. So in fact, this is rotating with a Larmor frequency. And there you go. In the same blackboard, you have a classical mechanics derivation of the Larmor frequency and a quantum mechanical derivation of the Larmor frequency. Again, at the end of the day, this is no coincidence. We've made dynamical classical variables into quantum operators, and we haven't changed the physics. Mu dot B is a classical energy. Well, it became Hamiltonian, and it's doing the right thing. So we can now use the Larmor frequency to rewrite the Hamiltonian, of course. It's here. So a little bit of emphasis is worth it. Hs is minus mu dot B, and it's minus gamma B dot S, and it's finally equal to omega L dot S. So if somebody gives you a Hamiltonian that at the end of the day, you can write it as some vector dot S, you already know that for spins, that is the Larmor frequency of rotation. It's a very simple thing. Hs, something times S, well that's precisely the rotation frequency for the spin states. They will all rotate that way. So we can say that the spin states in this Hamiltonian rotate with omega L frequency. So that's good. That's a general discussion of precession in a magnetic field. But I want to go one more step in generalization. It's a simple step, but let's just take it. So that you see even more generally why any system can be thought of as a spin system. And this is quite practical. In fact, it's probably the best way to imagine physically, the effects of any Hamiltonian. So let's consider time-independent Hamiltonians the most general Hamiltonian for a two-state system. How can it be? Well, a two-state system, remember two-state system is a word. It really means a system with two basis states. Once you have two basis states, a plus and the minus have infinitely many states, of course. But two-state system is two basis states. And therefore, in the Hamiltonian, in this two basis states, is a 2 by 2 matrix. And it's a 2 by 2 Hermitian matrix. So there's not too much it can be. In fact, you can have a constant that I will call maybe not the base notation, g not and g not. And that's Hermitian. It's real constant. You can put a g3 and a minus g3. That's still Hermitian. And that's reasonable. There's no reason why this number should be equal to this. So there are two numbers here that are arbitrary, real. And therefore, you can put them wherever you want. And I decided to call one g not plus g3 and one g not minus g3. Here, I can put again an arbitrary complex number, as long as I put here the complex conjugate. So I will call this g1 minus ig2, and this g1 plus ig2. And that's the most general 2 by 2 Hamiltonian. Tonya If those would be time-dependent functions, this is the most general Hamiltonian ever for a 2 by 2 system. It doesn't get more complicated. That's a great advantage of this. But I've written it in a way that you can recognize something. You can recognize that this is g not times the identity plus g1 times sigma 1 plus g2 times sigma 2 plus g3 times sigma 3. And this is because the Pauli matrices are, together with the unit matrix, a basis for all Hermitian 2 by 2 matrices. So the Pauli matrices are Hermitian. The unit matrix is Hermitian. The most general 2 by 2 Hermitian matrix is a number times the one matrix, then number times the first part, then number, second, number, third. OK. So at this moment, we've got the most general Hamiltonian. And I will write it as g not times 1 plus g vector dot sigma, where g vector is g1, g2, g3. If we write the g vector as length of g, which is just the letter g, shouldn't be confused because we have g not, g1, g2, g3, but we haven't had a g without an index. So g without an index is going to be the magnitude of g vector, and n is going to be a particular vector. So look, you're talking about the most general Hamiltonian, and you're saying it's most easily understood as g not multiplying the identity, and that g vector multiplying the sigma vector. So on the other hand, g is this. So this is also g not 1 plus g times n dot sigma. But let's continue here. We know how to solve this problem. And you can say, well, all right. I have to diagonalize this matrix, find the eigen vectors, find the eigenvalues, and all that. But you've done all that work. It's already been done. What were the eigen states? Well, n dot sigma, the eigen states were the end states, the spin states, n plus minus. And they were plus minus n comma plus minus. Remember that S is H over 2 sigma. So this corresponds to n dot S on n plus minus equal plus minus H bar over 2 n plus minus, which might be the form in which you remember it better. But the sigma matrices, n dot sigma is diagonalized precisely by this thing. So in fact, you never have to diagonalize this matrix. It's already been done for you. And these are the eigen states of this Hamiltonian. And what is the value of the energy on n plus minus? Well, energy on n plus minus is g not times 1 plus g n dot sigma on the n plus minus. And g not times 1 here on this state is g not plus g n dot sigma, the thing is plus minus. So plus minus g, n plus minus. So in fact, you have the energies, and you have the eigen vectors. So the eigen states are n plus with energy equal g not plus g and n minus with energy equal g not minus g. So what we did by inventing the Pauli matrices and inventing spin states and all that was solve for you the most general 2 by 2 Hamiltonian, Hermitian Hamiltonian. If you have a 2 by 2 Hermitian matrix, you don't have to diagonalize it by hand. You know the answers are this state. And how do you build those states? Well, you know what n is because you know the g's. If you know the three g's, you know what the vector g is. You know what the vector n is. You know what this g is as well. And therefore, with a vector n, you construct this state, as you know already very well. And given that you know g and g not, well the energies are this, at the splitting is 2g between those states. This is the ground state. This is the excited state. Splitting two g's, so you look at the Hamiltonian, and you say, what's the splitting between the two eigen states of this. You just take this numbers, compute g, and multiply by 2. Now, last thing that you would want to do with this Hamiltonian is time evolution. So what do we say about time evolution? Well, we have here H is equal to this. And we also had omega L dot S. So omega L dot S in here should be identified with this. So sigma and S, as you remember, S is equal H bar over 2 sigma. So this term can be written as g vector sigma. In fact, this is better from here. g vector sigma, and sigma is H bar over 2S. I got a 2 over H bar. 2 over H bar S. So from here, g dot sigma is 2g over H bar S. And remember, a Hamiltonian for a spin system, whatever's multiplying the vector that is multiplying S is omega L. So in this system-- I will write it like that-- omega L is 2g over H bar. And this is a great physical help. Because now that you have this, I should remark this part of the Hamiltonian is the one that does procession. A part proportional to the identity cannot do procession, is just a constant term that produces a constant phase, just produces a pure phase. That's a change, an overall phase that doesn't change the state. You would have an extra factor of e to the minus i times that constant, g not t over H bar, multiplying all the states. Doesn't change the action on plus or minus state. It's an overall phase. This term in the Hamiltonian is almost never very important. It doesn't do anything to the physical states, just gives them pure phases. And this term is the thing that matters. So now with this Hamiltonian, because g dot sigma is the form of the Hamiltonian, and we've identified this physical phenomenon of Larmor frequency, if you know your vector g for any Hamiltonian, this might be the [INAUDIBLE] for ammonia molecule, then you know how the states evolve in time. Because you represent the state. You have one state and a second state. You think of the one state as the plus of a spin, the minus of a spin. And then you know that this is processing with this Larmor frequency. So it may sound a little abstract at this moment, but this gives you the way to evolve any arbitrary state intuitively. You know the vector V where it points. You know where your state points in the configuration space. And you have a physical picture of what it does in time. It's always precessing. Therefore, the dynamics of a two-state system in time is always procession, and that's what we have to learn. So next time will be ammonia molecule, and then NMR.
Analytical solutions for stationary state energies and time-dependence
Representation
Supposing the two available basis states of the system are and , in general the state can be written as a superposition of these two states with probability amplitudes ,
Since the basis states are orthonormal, where and is the Kronecker delta, so . These two complex numbers may be considered coordinates in a two-dimensional complex Hilbert space.[1] Thus the state vector corresponding to the state is
If the state is normalized, the norm of the state vector is unity, i.e. .
All observable physical quantities, such as energy, are associated with hermitian operators. In the case of energy and the corresponding Hamiltonian, H, this means
The time-independent Schrödinger equation states that ; substituting for in terms of the basis states from above, and multiplying both sides by or produces a system of two linear equations that can be written in matrix form,
Of course, in general, commuting the matrix with a state vector will not result in the same vector multiplied by a constant E. For general validity, one has to write the equation in the form
One might ask why it is necessary to write the Hamiltonian matrix in such a general form with bra-ket-enclosed Hamiltonians, since should always equal zero and should always equal . The reason is that, in some more complex problems, the state vectors may not be eigenstates of the Hamiltonian used in the matrix. One place where this occurs is in degenerate perturbation theory, where the off-diagonal elements are nonzero until the problem is solved by diagonalization.
Because of the hermiticity of the eigenvalues are real; or, rather, conversely, it is the requirement that the energies are real that implies the hermiticity of . The eigenvectors represent the stationary states, i.e., those for whom the absolute magnitude of the squares of the probability amplitudes do not change with time.
Eigenvalues of the Hamiltonian
The most general form of a 2×2 Hermitian matrix such as the Hamiltonian of a two-state system is given by
Equivalently, this matrix can be decomposed as,
The Hamiltonian can be further condensed as
The vector is given by and is given by . This representation simplifies the analysis of the time evolution of the system and is easier to use with other specialized representations such as the Bloch sphere.
If the two-state system's time-independent Hamiltonian H is defined as above, then its eigenvalues are given by . Evidently, α is the average energy of the two levels, and the norm of is the splitting between them. The corresponding eigenvectors are denoted as and .
Time dependence
We now assume that the probability amplitudes are time-dependent, though the basis states are not. The Time-dependent Schrödinger equation states , and proceeding as before (substituting for and premultiplying by again produces a pair of coupled linear equations, but this time they are first order partial differential equations: . If is time independent there are several approaches to find the time dependence of , such as normal modes. The result is that
It can be shown that
When one changes the basis to the eigenvectors of the Hamiltonian, in other words, if the basis states are chosen to be the eigenvectors, then and and so the Hamiltonian is diagonal, i.e. and is of the form,
Now, the unitary time evolution operator is easily seen to be given by:
Rabi formula for a static perturbation
Suppose that the system starts in one of the basis states at , say so that , and we are interested in the probability of occupation of each of the basis states as a function of time when is the time-independent Hamiltonian.
The probability of occupation of state i is . In the case of the starting state, , and from above,
Obviously, due to the initial condition. The frequency
At zero detuning, , i.e., there is Rabi flopping from guaranteed occupation of state 1, to guaranteed occupation of state 2, and back to state 1, etc., with frequency . As the detuning is increased away from zero, the frequency of the flopping increases (to Ω) and the amplitude of exciting the electron decreases to .
For time dependent Hamiltonians induced by light waves, see the articles on Rabi cycle and rotating wave approximation.
Some important two-state systems
Precession in a field
Consider the case of a spin-1/2 particle in a magnetic field . The interaction Hamiltonian for this system is
It can be seen that such a time evolution operator acting on a general spin state of a spin-1/2 particle will lead to the precession about the axis defined by the applied magnetic field (this is the quantum mechanical equivalent of Larmor precession)[2]
The above method can be applied to the analysis of any generic two-state system that is interacting with some field (equivalent to the magnetic field in the previous case) if the interaction is given by an appropriate coupling term that is analogous to the magnetic moment. The precession of the state vector (which need not be a physical spinning as in the previous case) can be viewed as the precession of the state vector on the Bloch sphere.
The representation on the Bloch sphere for a state vector will simply be the vector of expectation values . As an example, consider a state vector that is a normalized superposition of and , that is, a vector that can be represented in the basis as
The components of on the Bloch sphere will simply be . This is a unit vector that begins pointing along and precesses around in a left-handed manner. In general, by a rotation around , any state vector can be represented as with real coefficients and . Such a state vector corresponds to a Bloch vector in the xz-plane making an angle with the z-axis. This vector will proceed to precess around . In theory, by allowing the system to interact with the field of a particular direction and strength for precise durations, it is possible to obtain any orientation of the Bloch vector, which is equivalent to obtaining any complex superposition. This is the basis for numerous technologies including quantum computing and MRI.
Evolution in a time-dependent field: Nuclear magnetic resonance
Nuclear magnetic resonance (NMR) is an important example in the dynamics of two-state systems because it involves the exact solution to a time dependent Hamiltonian. The NMR phenomenon is achieved by placing a nucleus in a strong, static field B0 (the "holding field") and then applying a weak, transverse field B1 that oscillates at some radiofrequency ωr.[3] Explicitly, consider a spin-1/2 particle in a holding field and a transverse rf field B1 rotating in the xy-plane in a right-handed fashion around B0:
As in the free precession case, the Hamiltonian is , and the evolution of a state vector is found by solving the time-dependent Schrödinger equation . After some manipulation (given in the collapsed section below), it can be shown that the Schrödinger equation becomes
As per the previous section, the solution to this equation has the Bloch vector precessing around with a frequency that is twice the magnitude of the vector. If is sufficiently strong, some proportion of the spins will be pointing directly down prior to the introduction of the rotating field. If the angular frequency of the rotating magnetic field is chosen such that , in the rotating frame the state vector will precess around with frequency , and will thus flip from down to up releasing energy in the form of detectable photons.[citation needed] This is the fundamental basis for NMR, and in practice is accomplished by scanning until the resonant frequency is found at which point the sample will emit light. Similar calculations are done in atomic physics, and in the case that the field is not rotating, but oscillating with a complex amplitude, use is made of the rotating wave approximation in deriving such results.
Here the Schrödinger equation reads
Expanding the dot product and dividing by yields
To remove the time dependence from the problem, the wave function is transformed according to . The time dependent Schrödinger equation becomes
Evaluating each term on the right hand side of the equation
The equation now reads
Relation to Bloch equations
The optical Bloch equations for a collection of spin-1/2 particles can be derived from the time dependent Schrödinger equation for a two level system. Starting with the previously stated Hamiltonian , it can be written in summation notation after some rearrangement as
Multiplying by a Pauli matrix and the conjugate transpose of the wavefunction, and subsequently expanding the product of two Pauli matrices yields
Adding this equation to its own conjugate transpose yields a left hand side of the form
And a right hand side of the form
As previously mentioned, the expectation value of each Pauli matrix is a component of the Bloch vector, . Equating the left and right hand sides, and noting that is the gyromagnetic ratio , yields another form for the equations of motion of the Bloch vector
As a final aside, the above equation can be derived by considering the time evolution of the angular momentum operator in the Heisenberg picture.
When coupled with the fact that , this equation is the same equation as before.
Validity
Two-state systems are the simplest non-trivial quantum systems that occur in nature, but the above-mentioned methods of analysis are not just valid for simple two-state systems. Any general multi-state quantum system can be treated as a two-state system as long as the observable one is interested in has two eigenvalues. For example, a spin-1/2 particle may in reality have additional translational or even rotational degrees of freedom, but those degrees of freedom are irrelevant to the preceding analysis. Mathematically, the neglected degrees of freedom correspond to the degeneracy of the spin eigenvalues.
Another case where the effective two-state formalism is valid is when the system under consideration has two levels that are effectively decoupled from the system. This is the case in the analysis of the spontaneous or stimulated emission of light by atoms and that of charge qubits. In this case it should be kept in mind that the perturbations (interactions with an external field) are in the right range and do not cause transitions to states other than the ones of interest.
Significance and other examples
Pedagogically, the two-state formalism is among the simplest of mathematical techniques used for the analysis of quantum systems. It can be used to illustrate fundamental quantum mechanical phenomena such as the interference exhibited by particles of the polarization states of the photon,[4] but also more complex phenomena such as neutrino oscillation or the neutral K-meson oscillation.
Two-state formalism can be used to describe simple mixing of states, which leads to phenomena such as resonance stabilization and other level crossing related symmetries. Such phenomena have a wide variety of application in chemistry. Phenomena with tremendous industrial applications such as the maser and laser can be explained using the two-state formalism.
The two-state formalism also forms the basis of quantum computing. Qubits, which are the building blocks of a quantum computer, are nothing but two-state systems. Any quantum computational operation is a unitary operation that rotates the state vector on the Bloch sphere.
Further reading
- A treatment of the two-state formalism, presented in the third volume of The Feynman Lectures on Physics.
- Lecture notes:
- from the Quantum mechanics II course offered at MIT, http://web.mit.edu/8.05/handouts/Twostates_03.pdf
- from the same course dealing with neutral particle oscillation, http://web.mit.edu/8.05/handouts/nukaon_07.pdf
- from the Quantum mechanics I course offered at TIFR, http://theory.tifr.res.in/~sgupta/courses/qm2013/hand4.pdf covers the essential mathematics
- http://theory.tifr.res.in/~sgupta/courses/qm2013/hand5.pdf ; from the same course deals with the some physical two-state systems and other important aspects of the formalism
- the mathematical in the initial section is done in a manner similar to these notes http://www.math.columbia.edu/~woit/QM/qubit.pdf, which are from the Quantum Mechanics for Mathematicians course offered at University of Columbia.
- a book version of the same ; http://www.math.columbia.edu/~woit/QM/qmbook.pdf
- Two-state systems and the two-sphere, R J Plymen, Il Nuovo Cimento B 13 (1973) 55-58
See also
References
- ^ Griffiths, David (2005). Introduction to Quantum Mechanics (2nd ed.). p. 353.
- ^ Feynman, R.P. (1965). "7-5 and 10-7". The Feynman Lectures on Physics: Volume 3. Addison Wesley.
- ^ Griffiths, p. 377.
- ^ Feynman, R.P. (1965). "11-4". The Feynman Lectures on Physics: Volume 3. Addison Wesley.






















































































































































![{\displaystyle i\hbar {\frac {d\sigma _{j}}{dt}}=\left[\sigma _{j},H\right]=\left[\sigma _{j},-\mu \sigma _{i}B_{i}\right]=-\mu \left(\sigma _{j}\sigma _{i}B_{i}-\sigma _{i}\sigma _{j}B_{i}\right)=\mu [\sigma _{i},\sigma _{j}]B_{i}=2\mu i\varepsilon _{ijk}\sigma _{k}B_{i}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2f9d54a643ad7c2b1ef3dd7934d6a7a372d02c1f)
